UNIFIED TABLE 2024 UPDATE

One sheet to track them all, one sheet to find them,
One sheet to sort them all, and in the data bind them,
In the depths of space where our worlds lie.

Hello dear visitor. It's been quite a while since I last posted anything on this site (over 9 months), so I thought that I could give you guys a little update on the model I use for all my writing and napkin calculations whenever prompted.

In case some of  you haven't noticed yet, HARD SCI-FI has a little link to a page containing all the calculators and spreadsheets I used for posts throughout the blog's history, it's on the right-side on the PAGES block.

It has been somewhat useful for me to revisit some of these tools from time to time in my creative process, but it can be quite overwhelming at times to open a dozen tabs and make copies of those as to not alter previous work. Back in 2022 I have attempted to summarize all the math used in my WIP guideline book (?), but as of them it was rather incomplete, and as of now it does not reflect my current understanding of its aims and the science of it.

 

Screenshot of the 2022 table, on STARS

So now, until the end 2024 I'll be working on a new table of contents that summarize the math in each of the chapters for ease of use, it will be also be a more consistent model as now the different tables will be able to cross-reference themselves, for a reasonable  approximation of reality as I've come to know it.

Though, I'll likely keep the same  streamlined look and palette to it.

Also, leaving this disclaimer from the opening text from the guide (before anyone comments on it):

Therefore, this work establishes a set of rules for a simplified model of the universe, which, while similar, is not precisely like our own.

- M.O. Valent, 17/10/2024 

SCIENCE&ARTWORK | BINARY STAR SUNDIAL | PART 2

WHAT ABOUT DIFFERENT STARS?

So excited Nyrath from Atomic Rockets shared my last post! LETSGOOO

A few reddit users pointed out if the bisolar clock I designed assumes that both stars are the same mass, thus orbiting a common center of mass equally spaced between them, here is why I made this initial assumption while working on the model:

So as you can see, we've got a bit of a problem here, not only with getting the instant time of the day when the two stars are not of similar mass, but also getting the time at any point in their orbital period, since this geometric center would wobble back and forth.

However, the greater the mass difference, the greater the difference in luminous output from the stars, so in a way - the greater mass difference would make the secondary component less relevant for the clock building.

1.0 Msol ~ 1.00 Lsol

0.8 Msol ~ 0.50 Lsol

0.5 Msol ~ 0.10 Lsol

0.2 Msol ~ 0.01 Lsol

and so on...

So with greater mass difference, unless we're dealing with massive stars like F or A-types, the less relevant the secondary component becomes, but luminosity follows roughly the cube root of the stellar mass, which means that although the luminosity from the secondary isn't as influential, its mass still is. That dim star would still make the brighter component wobble.

From the given examples above, the 1:2 system has an orbital period of 41.17 days, and the 3:1 of 43.66 days - the 1:1 system is the same as the previous post. The secondary in each of the two examples has luminosity as 0.06 and 0.02 Lsol, quite insignificant for any clock building purposes, as the primary is about 10x as luminous.

Since both systems have similar luminosity, I will set up our observer planet at 0.75 AU for both. The maximum elongation of the primary is then 6.35° for the 1:2 case, and 4.74° for the 1:3 case.

If we keep the 36 hour day, that means our clocks may be up to 38 and 28 minutes ahead/behind barycenter time. And since this difference develops slowly over the course of some...

1:2 case ~ 50.97 days, planet year is 213.8904 Earth-days,

1:3 case ~ 54.32 days, planet year is 226.8933 Earth-days,

We can just add those variations to the equation of time, by adding a sine wave with the equivalent time difference amplitude and synodic period of the stars. I'll use 20° axial tilt and 0.01675 eccentricity for the EoT generation.

1:2 case

1:3 case

Given this solution, any ordinary Earth sundial works in such world, for as long you consider this modified equation of time for your planet, just look for the darker shadow whenever checking the time.

KEEPING TIME AROUND AN 8-SHAPED ORBIT???

Another user spoke about a project they have involving a planet with a 8-shaped orbit around its two parent stars, with a pretty usual set of circumbinary planets after it.

Now, since it is not the goal of the project to be 100% Interstelar-level physics plus some magic dust sprinkled on top, I'm not getting into how to plan those orbits, or how no planets could form under such conditions, let's just exercise and have fun, because some realities are just sad.

You might also want to look into Arenstorf Orbits - those can be pretty dope and are used for planned satellite missions. About the 1 year period, I'm rather unsure if that's possible at all without having the stars several AU apart (and the planet freezing midway to the other pair), the proximity to the stars and orbital speed necessary for orbital transfer inside the gravity well of whole stars would mean that the planet likely going to complete the circuit in a few days or even hours, in what it is called a Free Return trajectory.

In this situation, no conventional methods for time keeping work, because sundials work on the regular passage of the sun, and it gets even worse if one actually opts of an Arenstorf-like orbit, where you've got many loops of different sizes. Given that, it seems more efficient to track the time at night, when the stars are visible - if the planet rotation happens within reasonable fraction of the planet orbital period, say like, within 7 local days, or if the planet somehow rotates at such speed that a single hemisphere always faces forward in the orbit, and the other trails behind.

At different points of the orbit, the night/dark sections of the planet would point towards different constellations of the local zodiac, ex; some constellations appear on the east only if you're approaching the smaller stars, while the same constellation appears on the west as you leave that star - because the planet is now facing the other direction.

As the stars orbit each other over a period of less than 100 days, those constellations would change as well (given sun-like stars at < 1.0 AU), returning as they were after some 3 months. Plus, given the planet rotation being similar or even longer than its orbital period, we get some trippy sun movements, I suppose even more confusing around two stars.

For timekeeping during the day, there is one simple scheme I propose, a pinhole projection clock!

As the planet races from one star to another, the apparent size of the stars in the sky changes, and a safe way to measure this is through a pinhole projection, since the projection size is always proportional to the object's true size in relation to its distance from the observer.

R/D = r/d

Where R is the real diameter and D the real distance, and the r is the projected diameter and d the distance between pinhole and screen.

By tracing concentric circles of different sizes inside the box, one can know the precise time in terms of the planet's trajectory around the suns - like quarter, half-way, and maximum diameter for one or both stars, and all you have to do is to point the box and look inside through a lateral aperture. People have used a very similar setup to watch solar eclipses here on Earth.

With the invention of glass and lenswork, a more compact and sophisticated method can be used, using a small telescope or lens to project onto a small screen (I imagine such an instrument would be size of a toothpaste box), and the same concentric circles inscribed in the screen would serve to tell the time down to the day.

UNTIL ANOTHER DAY!

- M.O. Valent, 09/03/2023

SCIENCE&ARTWORK | BINARY STAR SUNDIAL | PART 1

IS IT POSSIBLE TO CONSTRUCT A BINARY STAR's SUNDIAL?

WHY?

So this last week I've been trying to work on my own sundial to settle up an argument (with a flatearther, ugh), It works pretty nicely and got bit damaged by rain since it is made of paper, but my brief study of Gnomonics got me pretty interested in the craft as a whole, there are 'easy' and 'hard' ways to make sundials and solar calendars. You can scroll right to the end if you're not interested in the whole thought process behind its end design.

My first print of my gnomonic sundial, the lines are created by projecting a spherical grid onto a plane which is the dial plate. 

Anyone can use a base and a stick to simply mark the hours by the gnomon's shadow, but the instrument will only be able to tell hours. But to be able to tell the day and months/seasons, the designs and matemathics get a little bit more complex.

Fellas over the northern hemisphere might already be familiarized by the sundial design above, formed by a disk or dial plate, with a triangular gnomon on top of it. None of the shapes here are of arbitrary dimensions, the inclination angle of the gnonmon has to match your Latitude (North/South coordinate), and the size of the gnomon in relation to the plate will determine wether the instrument can only tell hours or include the seasons/month markings. For the northern hemisphere inhabitants, one can eyeball the inclination of the gnomon by pointing to Polaris, the North Star, whereas southern people have to use known landmarks and constellations to get a sense of where to point it.

If you look at my design, you will notice a straight line, and two opposing hyperbolae, the straight line is called the Line of Equinoxes, it is where the shadow of the gnonomon falls during the Autumn and Vernal equinox days (Equinox or Equinoctis meaning "equal nights") - the two opposing hyperbolae are the Solstice lines, where the shadow falls during the summer and winter soltices respectively, by marking intermediary hyperbolae one can mark the passsages of months and weeks through the solar cycle.

Because of the Earth's tilt, one can draft a simple grid like the one I did by constructing a spherical grid marked 23.5° north and south of the equator, then putting a light source at its center, then tilting said spherical grid to the user's latitude - the resulting projection onto a sheet of paper will be your markings, and the distance from the paper to the center of the sphere is the same from the plate to your gnomon's tip, which is what you can see I rendered in the 3D software, then printed.

Without a 3D grid (physical or virtual), it takes a lot of math to traditionally work out the specific lines you'll need for you sundial, that's why the ancients often cut the middle-man and just projected their sundials onto convex hemispheres and rings!

There is a whole bunch of other types of sundials each specific to what their projectionists wanted to be told by the sun as well, but do any of those work for a world with two suns?

THE SETTING

The system's stats I will be using thoughout this post

First of all, a quick googling of "Binary Star Sundial" retrieves not a lot of material, if someone has ever worked on anything like that, they didn't publish it on the internet, there are a couple posts on stackexchange and reddit dating back to 2016 but no solutions, most of them assume you can get away with a normal sundial since the shadows created onto a circumbinary planet would mostly be off by about 10° maximum - but this would only work as an approximation, depending on the specifics of the system, this would mean the clock could be offset by about 1h of the actual time (assuming the planet has 24h day/night cycle).

So before tackling the problem as a whole, let's see what changes from the traditional Terran sundial, so we can better know what challenges lie ahead:

DIFFERENCES BETWEEN SOLAR AND BISOLAR CLOCKS

1. MOVING SUNS

In the traditional solar clock, one can assume an inertial reference point, that could be either the static Earth with the Sun moving around it, or the static Sun-Earth system, with the Earth rotating around its axis.

On a bisolar clock, we have to consider not only either of those scenarios, but also that the suns are not static in the sky, they revolve around a common center of mass. Which means that for either scenario, the suns would always move significantly on a day to day basis - what would in itself, create a solar subcycle our natives to work with.

2. MISALIGNED ECLIPTIC

On Earth, the Ecliptic plane is the apparent path of the Sun across the sky throughout the year, it is pretty easy to follow through and it is what defines the constellations of the zodiac. On a circumbinary system however, one could either define the ecliptic as the planet's orbital plane, or the star's own orbital plane, in either case, both stars would constantly fall above and below the ecliptic as they orbit each other. Which means that the difference in orbital inclination between the stars and observer planet would change throughout the year as well. Anyone familiar with tracking the movements of Mercury and Venus in the sky will known how crazy the paths can look.

Because all of those movements are specific to the times and cycles at play, I will outline my process with an example so you can work out your own models of bisolar clocks. Since we cannot experience such a place, I will be using 3D software to simulate what it should look like based on the model stats given in my sketch.

UNDERSTANDING THE SKY

To keep things simple, I will work with geocentric coordinates, swapped the Sun in the solar system by a pair of twin stars with 80% of the mass, just so the brightness matches just the same on our planet, which had its tilt reduced to 20°. The star's also orbit each other at an angle of 5° from the planet's orbit.

Although for an external observer, the twin suns orbit each other every 36.1 days, the planet which is also orbiting the stars do not perceive this as being 36 Earth-days, we need to calculate the synodic period of the stars by using:

Which works out to be 41.25 Earth-days, or 990 hours, now we can use this information to set up the planet's rotation into some neat value that's easier to work with.

I will set the planet's rotational period to about 36 hours, this gives us a simple 10° per hour rotation, it approaches the solar subcycle to roughly 27.5 sidereal days (similar to a lunar month) - and makes the year about 192½ days. Meaning our stars move 13.1° over the course of a day, and half as much during daytime.

corrected suns declination at +0.624°

Usually, the planet's tropics would be located at latitudes 20° North and South of the Equator, but because the suns can gain about 0.62° of declination along their orbit, we can say that the tropics are found between 19° and 21° from the equator. This means that depending on the suns-planet alignment, the extra declination can come during solar opposition (suns seem aligned) or during maximum elongation (suns seem further apart).

The maximum elongation or separation between the suns in the sky would be about 14.5°, and this gives us another interesting alternative to measure the solar day, Mean Solar Time, which is measured from the barycenter, considered static while the suns orbit around it.

Further refinements put the sidereal day at 35h52m33s for a perfect 36 hour solar day

Let's also start our year in a point in the orbit when the suns seem further apart.

DESIGNING THE HOROLOGIUM BISOLARII

PRELIMINAR EXPERIMENT

An anallematic sundial consists of a vertical gnonom which marks the time throughout the day, but also tracks the months through the solar anallema by the length of the gnomon's shadow.

On Bisolaria, such an anallematic clock would produce two shadows which dance with twice the speed of the suns orbital period.

Each square is 10cm wide for scale, and the gnomon has 10cm from the base to the center of the sphere, the star light was tinted yellow and blue to differentiate, each time they switch sides corresponds to half a solar subcycle, and the whole animation takes place over two years (~385 sidereal days) on this planet, Bisolaria.

Each frame in the animation is taken at exact mean solar noon - if we picked a star to count solar days, then the frame of reference would wobble back&forth across all the four cardinal directions as that sun moves across the sky throughout the year, undesirable, which is why we count from the barycenter. Here we can also see the effects of the star's orbital inclination, at times a shadow appears longer than the other by a noticeable amount.

I bet we can use that tilt in the stellar orbital plane and the fact we are working with two shadows to come up with a creative horizontal anallematic clock and calendar. Why not double down with two gnomons then? My initial idea is to use two longer gnomons spaced in such a way that their shadows cross most of the time, the line at which they cross would determine the time of the year, while the point at which they cross would make up for the current hour of the day.

LOOKING AT THE SKY

So throughout the years, we would see the suns eclipse each other only during the planet's passage through the nodal line, where the sun plane crosses the ecliptic plane. We would also see the sun plane appear to tilt north or south as we cross the south and north pointing sides of the orbit, which by itself would be a good teller of seasons.

But we also have to consider the effects of the planet's orbital eccentricity, because the planet moves faster around the suns when it's closer than when it is farther - this causes the mean solar time to go faster and slower than the planet's true rotation period by a few minutes, which is what the Equation of Time is about.

I'm not mentally stable nor know math enough to digest the needed equations so I could explain them better, but I've found a couple tools online that will be useful in generating the data require to produce a clock for a fictional world:

• Analemma Calculator
• Sage Cell - Equation of Time

While the Analemma Calculator is pretty straightforward with single-sun systems, we'd need more work for the paths of the two or more suns we include. Below is a generalized version of the SageCell code, pointing where you need to input your data - if you're not familiar with Earth's parameters.

OTHER | SEEKING CREMATORIA-LIKE WORLDS | PART 2

PREVIOUSLY, ON HARD SCI-FI...

We want a planet that:

Is somewhat habitable, but only if lighting conditions are just right, that is - it has an overilluminated side and a dark side. In which case, life would need to move or cover itself when the surface passes through the overilluminated side, and do its thing when it is dark until day comes again. Like Crematoria from Chronicles of Riddick.

EXPLORING WAYS TO MAKE CREMATORIA REAL:

From the previous post...

• Trojan planets around single stars
• Trojan planets around binaries

 Now exploring...

• S-type planets in close binaries
• Dying binaries
• Luminous black holes

LIFE BETWEEN TWO SUNS

Since we've already discussed how binary systems work in the previous  post, let's jump straight into a sketch of my plan for this setting:

Now, although in my sketch the stars are set in a 1:1 mass ratio, that will definetly not be the case, both for being a lone binary, and because we will need a generous safe zone around our planet's orbit in order to have minimum gravitational disturbing from the other star.

Since our planet needs to be sufficiently illuminated by the other component, we can already infer it lives within a close binary, which means we will have other P-type planets in the system as well, and this world would be actually a captured planet by one of the pairs.

If we make the parent star sun-like and put the planet in the habitable zone, then we would have to put the other component at +3 AU away, which is observationally bad for us since the illumination of the secondary pair would drop down to <10% of the solar constant.

But if the parent star is small, such as a red dwarf or orange dwarf, we can put the other brighter star much closer, it also helps that we get a significantly larger planetary disk around stars with mass disparity like that. In that case we will explore two sub-scenarios, in which this mass ratio is 3:1 and 2:1, the most common mass ratios for binaries.

3:1 case, system must be smaller than 0.26x the orbital separation

A ~ 1.00 Msol, 1.00 Lsol, 1.00 Rsol, 5778 K - G2V yellow dwarf

    Mean HZ for A: 1.00 AU

B ~ 0.32 Msol, 0.03 Lsol, 0.40 Rsol, 3800 K - M0V red dwarf

    Mean HZ for B: 0.21 AU

Orbital separation set to be at least 4x that of component B's habitable zone, or about +0.80 AU.

With minimum requirements, the solid airless surface of the planet would be at 310 K or 37°C, but we can cool it down to about 20°C if the parent star orbits the brighter star at a distance of 0.93 AU. Slap it a moderately thick atmosphere at 0.5 bar for an Earth-sized planet, and we got ourselves a simmering 50°C of surface temperature, with some 70°C at the equator, for a 24 hour rotational period.

That average temperature will ocillate between 32°C and 79°C as the planet orbits and points towards or away from the brighter star, changing irradiance between 1.47x and 2.63x the solar constant. These changes occur very quickly over the course of 66 days, but the planet is tidally locked to its parent star, making it a seasonal eyeball planet.

If we want to make it so it is not tidally locked, then the we can place it a bit further away at some 0.30 AU, decreasing the average temperature from 50°C down to 33°C, with an average irradiation of 1.50x the solar constant. Ocillating instead between 4°C and 87°C.

Because our red dwarf parent is quite large, we don't have to worry about sudden violent X-ray and UV flares even though we have a thinner atmosphere than Earth does. Plus, given we know the Earth's atmosphere cools at roughly 0.5~1.0°C per hour (depends on place, at my region it is roughly 0.8°C/h), we can tune the planet's rotation period to cool down to an acceptable level before heating up during the day, so if we want the temperature to drop from some 60°C during the day, down to 20°C at night, we'd have to set night-time duration to some 50 hours, which means our planet should have a rotational period of at least 100 hours to have decent cooling.

The rotational period of 100 hours bring the equator temperature to some 50°C as well, which means that during the night we have it dropping to 10°C. But that's only on the side of the planet which faces the two stars at once, we might not even have enough exposure time to hit some 70°C for most of the year. When the planet finds itself between the two stars the brighter side heats may cool down to some 14°C on average, and the dimmer side to a freezing -60°C. Because of the planet does not stop in this position for long, these potential extremes might not be reached, instead staying between well 70°C and -60°C in the equator across the whole orbit.

But temperatures might actually be stable and comfortable towards the planets poles given the softer ever setting suns or just by being in a temperate zone, though in general the whole planet's temperature changes drastically over the year.

We should also give it vast oceans, so we have ices and decent temperature buffers, so we don't end up with a venusian planet. Now, depending of the latitude, one will still get hot deserts during the day and freezing cold nights, and one half of the planet will always be considerably colder than the half facing the brighter star. So far, it seems we've accomplished our goal with moderate success.

After days in the dark, the brighter sun finally rises, thawing glaciers and lakes to form rivers which will flow fresh for the next two days until it sets again. Clibanus' thin atmosphere allows for stars and the system's outer planets to be observed even during the day, despite the radical changes in irradiation, flooded plains allow consistent liquid water for life

Compared to the original Crematoria, unless the atmosphere can dissipate the heat twice as efficiently as the Earth does, the planet of the movie cannot have 52 hour days, not can have the extreme temperature differences we see in the movie from 370°C to -180°C.

By default, this arrangement is pretty common and actually pretty likely to occur in nature, HOWEVER one thing I've stated at the start is that given  this planet is very close to the secondary star, it is possible that it is a captured planet, thus it might also sport other quirks such as high orbital inclination, high eccentricity, retrograde motion as well. The high eccentricity in particular seems like an interesting way to vary the temperature extremes even more, as well as varying the amount of water present in the planet's climate system.

For planets in more circular orbits, ie, that formed around the red dwarf, we are limited in how massive we can make that planet the same way we are limited with gas giant moons, in this case topping between 2 to 10 Earth-masses, which we could actually distribute in a planetary system of Terran and Subterran worlds which suffer from the same condition, but varying climates according to their specific atmospheres, water content, and distance from the red dwarf.

2:1 case, system must be smaller than 0.22x the orbital separation

A ~ 1.00 Msol, 1.00 Lsol, 1.00 Rsol, 5778 K - G2V yellow dwarf

    Mean HZ for A: 1.00 AU

B ~ 0.50 Msol, 0.10 Lsol, 0.57 Rsol, 4334 K - K6V orange dwarf

    Mean HZ for B: 0.39 AU

Now because our habitable zone around the secondary component expanded to double the previous orbital radius, we have to change the distance between the stars just a bit to accommodate those changes. So the system's minimum size, given the planet has to orbit within 22% of the orbital distance, is about 1.82 AU, orbiting every 2 years. This way, the star's hill sphere is about 0.8 AU in radius, snuggly fitting our habitable zone at 0.4 AU, and given our parent body is larger than the previous one, our planets can be up to 6 Earth-masses in size, even giving space for superterran worlds in orbit.

At such distances from the stars, the planet gets 0.63x and 0.30x the solar constant from its parent and the brighter star, respectively, being close enough to barely rotate instead of being tidally locked. Its equilibrium temperature is around -24°C, and it warms up to 10°C under 1 Earth-atmosphere. By setting the planet's rotation to 500 hours the equator is able to heat up to some 23°C.

When the planet is between the stars, the orange side drops down to -17°C and -4°C on the equator, while the yellow side to some -50°C.

We can already stop right here, as it was concluded from the last example and from the last post that trying larger stars help, but not a lot because of the greater distances involved.

DYING BINARIES

It is not (observationally) uncommon for a bright star to have a dead or almost dead companion in a binary pair, see the Sirius system, an A-type white star and its white dwarf companion Sirius B. However, since the Nova which forms a white dwarf most likely destroy the planets around it (if any survived the red giant phase) is too dim to provide any significant illumination, we will be looking at red giant pairs.

If we want planets in those systems to be habitable for a long time, then such systems would have to be either very old and originating from sun-like stars, or very young containing a sun-like star and a heavier pair. Whatever the case we choose, it will be composed of a star still in its main sequence, and a bright red giant. For the distances we can be somewhat liberal, alerting only for the absence or presence of planetary disks. Let's say that by the time our primitive planet starts developing complex life, our heavier star finishes its MS phase and starts diving into the red giant phase. A sun-like star spends two billion years in the red giant phase, with variable luminosity as time passes, very variable in the last few hundred million years, which means such worlds are pretty ephemerous. Let's use that as a basis, with a star that enters the sub-giant phase at the age of 4 billion years, setting the system's age half-way through the process. The chosen mass ratio is 5:2, still close to your typical common binary system.

A ~ 1.28 Msol, 2.47 Lsol, 1.22 Rsol, 6566 K - F5V yellow-white star (nominal stats)

Mean HZ for A: 1.58 AU

SUB-GIANT STATS:

Current Age: 4.48 Gyr, 400 million years until the Helium Flash.

Current temperature: 4000 K, it will continue to drop to 3000 K until the Helium Flash. 

Current luminosity: 5 Lsol, it will continue to steadly rise up to 25 Lsol, before taking off to 2500 Lsol for the Flash in a short period of 100 million years.

Current radius: 2 Rsol, it will steadly rise to 10 Rsol, until inflating to 200 Rsol (1.9 AU) when the Flash happens. 

Mean HZ for L ~ 5.0 and L ~ 25.0: 2.2 AU and 5.0 AU

B ~ 0.50 Msol, 0.10 Lsol, 0.57 Rsol, 4334 K - K6V orange dwarf

    Mean HZ for B: 0.39 AU

Just so we keep the secondary pair, and thus the planet, under a significant illumination from the dying star, the orbital distance will be about 3 AU. So as the ages pass, the planet will go from receiving 0.5 to 2.8 solar constants from the dying star, while getting a consistent 0.6x solar constants from its parent, so the total illumination will rise from 1.1 to 3.4 over the course of 400 million years. Under 1 Earth-atmosphere, the mean temperature rises from 22°C to 105°C over this period, staying about 20 degrees hotter than those in the equator. The coldest tropical nights in such a world simmer at 65°C, while the planet is more temperate towards the tropics, however, with such high temperatures, we need to make this a waterworld to buffer it all, or a dry rock so the clouds don't start a runaway greenhouse effect. We could also balance the temperature by smaking the planet smaller, which decreases air pressure and volcanism so we don't turn this world into a venusian right away. A half as thin atmosphere puts the planet on pair with the previous world even on the hottest phase of its life.

By varying the amount of water in the atmosphere and a little bit of the secondary star's eccentricity around the main component, we can better control the surface conditions, still obeying the same principles as before, though we now know such worlds will be flash burned when the subgiant becomes a red giant, elevating the mean temperature to about 850°C.

Given what we know from how atmospheres react to the incoming light of different stellar spectra, it is safe to say that once the insolation coming from the red giant exceeds 0.7~0.8 solar constants, the planet will be no longer habitable, for the amount of infrared the atmosphere receives can no longer be irradiated away to space efficiently, thus, the surface temperature rises several dozen kelvin for even the tiniest increase in luminosity.

Reproduced from Habitable Zones around Main Sequence Stars, JF Kasting et al, 1993

We can say for sure that our planet around a red giant won't be habitable for much longer than half of its life between main sequence and the helium flash.

Hence why whenever I set up a planet around red dwarfs I try to keep their insolation below 1.0 and above 0.5, one can go even lower if they wish to add brighter stars to the system, if those stars are considerably hotter, even a few percent more incidence will the job at illuminating and heating the planet up without tipping it over the edge of a runaway greenhouse event.

Though I admit a future me or one of my readers will eventually find something I overlooked or winged for sake of argument for this post series, after all, this is supposed to be an expositional guide, an exercise for you to explore other particular variations more invested than I was with those quick examples.

DO NOT GO GENTLE INTO THAT GOOD NIGHT

Ah yes, luminous black holes and their blanets (yes with a B, that's a thing). For the ones not familiar with the concept, it's the kind of scenario as presented in the movie Interstellar (2014), but for the ones not familiar with the inner workings of such systems, I must warn such systems in nature would be so rare and ephemerous that one might as well regard them as legendary oasis, from what I could find and understand.

WHAT KIND OF BLACK HOLES ARE SUITABLE?

Black holes are a very interesting class of objects for their extreme variety of sizes and surrounding structures. The smallest black holes are created by stars above 23 solar masses, when their core collapses at the end of their lifes, compressing a good chunk of its matter into an infinetly dense point, at least some 2.9 to 3.0 solar masses, the infalling material and subsequent radiation burst ends up bouncing back up and eventually spewing all of the star's upper layers away into space, hence why the resulting black hole is rather small compared to the star's mass. Such small black holes are called Stellar-mass black holes, for they have the mass of typical high-mass stars, and typically, those black holes are the most dangerous for civilizations, for they are very small to the size of a few dozen kilometers, zipping through space at stellar speeds, gravitationally interfering with other systems as they fly by, and worst of all, they are often very dark - because the are rather small, it is very easy for matter to orbit and catapult around it without getting even a tiny bit close enough to be shredded or absorbed into it, thus they rarely emit or posess detectable signatures but the light-bending their gravity produces.

Stellar black holes are also much more aggressive than heavier ones for their small size, with a virtual density much higher than massive black holes, that is, the density you would expect if it was a solid object the size of its event horizon - a person standing 100 event horizon radii from a 5 Msol black hole would experience a gravitational gradient between their head and feet of ~750m/s, leading to instant material failure of the astronaut and resulting spaghettification. Whereas this same configuration with a 1000 solar mass black hole causes a difference of only 0.02m/s, far more tolerable.

Massive black holes are gentle giants.

Larger stars produce large black holes nearly twice the mass of these, but since stars rarely exceed masses above some 50~100 solar masses, stellar black holes cannot get any bigger than some 10 solar masses. From this point onward things get strange, black holes can only get bigger by absorbing lots of matter and other black holes, which means that the larger black holes are often much ancient than most stars, planets, or even the galaxy it currently inhabits.

When we look at massive black holes and supermassive blackholes, those which range from thousands to millions of times the mass of the Sun, we often find those which are surrounded by large disks of infalling matter, accretion disks. As matter accelerates towards the black hole, it rubs against other infalling molecules, heating up to thousands of degrees, generating all sorts of radiation, including light - those are the Luminous black holes. Non-luminous black holes include the ones such as Sagittarius A* at the center of the Milky Way, with 4 million solar masses, it has barely any accretion disk, existing in the dark, puppetteering nearby stars around a seemingly empty region of space. The feeding rate of a black hole, or accretion rate, is limited by its Eddington accretion limit, which is how much mass can fall into the black hole, before the resulting radiation pressure of the accretion disk counteracts the gravitational force of the infalling matter, the brightest luminous black holes such as quasars, blazars, and young radio galaxies find themselves near this limit or at super-Eddington limits, when the black hole also absorbs the extra radiation it would emit despite greater accretion rate. For obvious reasons, blanets and stars cannot reside near such monsters, because they would quickly be disintegrated into the accretion disk.

However, one detail we have to pay attention to while looking to settle black holes with habitable conditions, is that for most of their life, black holes will exist in their dark form, while luminous black holes are rather ephemerous. A single black hole might go through several luminous phases along its life, feeding on unlucky stars for a few million years, then waiting in the dark for the next prey, hence why radio galaxies are always young, as their central black holes did not have enough time to clear their surroundings, so not yet in their dark phase.

The amount of radiation released from a luminous black hole is directly proportional to the infall of matter, sometimes a black hole will traverse a region of space with little more gas than usual and shine very dimmly with a ghostly echo, or sometimes a whole rogue planet falls in, quickly spaghettified into a bright accretion disk which, like the rings of Saturn, will last a few million years.

Because we want blanets, moons, maybe even other stars around our luminous black hole, the gas around it which is the precursos to all of these bodies will be likely of solar-composition, with some sprikle of metals and not only hydrogen gas like the interstellar medium. The black hole would have to be near the end of its feeding / luminous stage, as we still want an accretion disk as energy source, but not so large of an accretion disk it just disintegrates any rocks with dense x-rays. So if the Eddington limit says the max accretion rate is a few billionths of a solar mass per year, then we will lean towards trillionths of a solar mass per year.

Because the surface area for an accretion disk around such black holes is immense, many times that of whole stars, the surface temperature of the disk should be star-like, between some 6000 to 2500 K, this works out to quite a headache of math when you're not familiar with the principles or equations behind it...

THE GENTLE GIANT

For an Interstellar-like scenario, we'll use a supermassive black hole about 100 million solar masses, spinning at 99.995% the speed of light, an ancient monster which hasn't fed upon anything for many millions of years, just now licking the breadcrumbs of its plate, that is, with a very thin ghostly accretion disk.

We're talking a 1.0 AU radii event horizon, with a disk that extends from 1.3 to 2.5 AU.

Our Eddington luminosity is around 4~6 trillion solar luminosities (depending on the gas makeup), with an accretion rate of 2 solar masses a year. So if we want the disk's Earth-like insolation zone to be at around 3 AU from the monster, we need an effective luminosity of 9 solar luminosities. So now we divide 9 by 4 trillion, we then get 2.25 trillionths of 2 solar masses, or 0.00012 Moon-masses a year. With a temperature between 288 thousand K near the ISCO down to 83 thousand K near the edge.

Even though the temperature is not enough for hard X-rays to be emmited through the ionization of metals within the disk, most of its emissions are still in the far UV spectrum. This can be avoided by increasing the opacity of the gas, making it partially ionized in a wider disk or toroidal cloud around the black hole. This makes the inner rim of the disk extremely hot while keeping the outer parts of the disk less hot, which means we need to lower the metal content of our gas cloud, or else the breaking radiation of relativistic electrons will increase the x-ray output of our accretion disk.

As for planetary formation around such objects, it would boil down to general rules of planetary formation, except the progenitor gas cloud would be the spewed guts of one or more stars devoured by the black hole, which for our purposes would have to be the black hole's last meal in a long time, or else the extreme x-rays would just photo-evaporate our blanets.

Those conditions will be very rare or even impossible to accomplish in real life, like, even a small rogue asteroid coming from interstellar space and falling in would increase the disk's luminosity by orders of magnitude - frying whatever life existed in the blanet surface. In the whole universe with its countless blackholes there might exist very few of those legendary oasis where conditions are just right, where life is possible hanging from a silk thread.

Because photon-matter interactions are rather too complex to bother going through, I'd admit handwaving most of them away would be the best course of action - for the sake of story telling, the habitable zone distances and time dilation regarding proximity to the black hole would have way more weight to it.

But realistically, given the many unknowns regarding radiation tolerances, a habitable blanet would look like the following:

Assuming the event horizon is some 10° wide in this image, the planet would find itself at 6 AU, receiving 1/4th of the Earth's insolation.

Would that be enough? I don't know, my IR correction equations are calibrated for stars, not massive accretion disks, the output for this case is right at the face of the event horizon inside the disk's inner radius, at 1.2 AU

An icy/oceanic superearth far far away from the black hole, some 10 Earth-masses and between 2.0 and 2.5 Earth-radii, the illumination is pretty dim compared to Earth's, but the incidence of x-rays and electron wind against the thick hydrogen/helium rich atmosphere reacts to produce scattered radiation, which warms it up to a tolerable temperature between 200 and 400 K. The atmosphere however would be rather anoxic, as the rays cannot penetrate very deep to react with the water or ammonia which pools on the surface as oceans, and any bacteria that develops here would be anaerobic, feeding on high energy or infrared light rays and minerals dissolved in the oceans, in a way, similar to Miller's planet - except much dimmer, much redder, and warmer.

For flavor we could add our habitable planet as a moon of a gas giant, or the more unlikely case - as the tidally locked planet of a red dwarf, working pretty much the same way as the first example from the start of the post, which seems to be the only viable way to obtain the desirable Crematoria-like effect

- M. O. Valent, 13/02/2023

OTHER | SEEKING CREMATORIA-LIKE WORLDS | PART 1

CAN THE SUN BE A DEADLY LAZER?

Alright, let's establish some objectives with the rush of ideas, we want a planet that:

Is somewhat habitable, but only if lighting conditions are just right, that is - it has an overilluminated side and a dark side. In which case, life would need to move or cover itself when the surface passes through the overilluminated side, and do its thing when it is dark until day comes again. Like Crematoria from Chronicles of Riddick.

We will explore different scenarios in which that world might exist, and rank the possibilities from Natural / Realistic down to Designed.

One first idea that came to mind was to add more stars to the system, and organize them in such a way as to cause the desired effect... Can it be done?

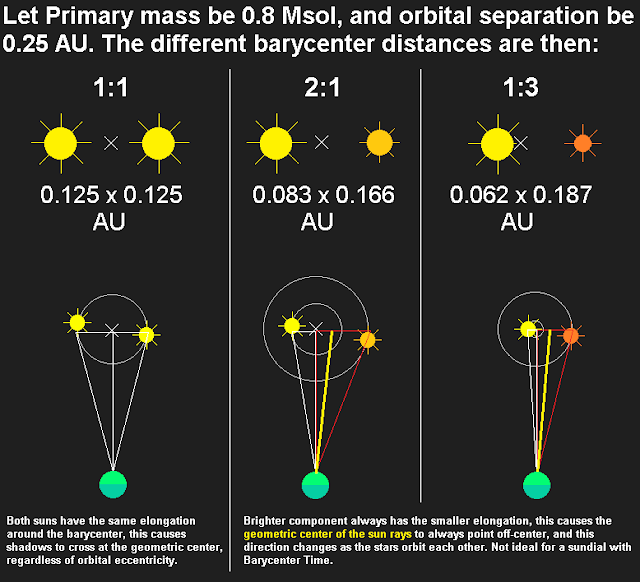
RECAP ON DOUBLE STAR SYSTEMS

The illustration above sums up a simple case and a generalization of the conditions in which protoplanetary disks form in binary systems. Here we see that circumbinary disks occur only when the orbital separation is around 3-ish AU or less, and we don't see any disks around either star up to at least 50 AU separation between them - this occurs because given eccentricity of the system, no sufficient mass might be able to clump into a protoplanetary disk either because the stars scattered it all around a circunstellar cloud / off-system, or because all of the mass was consumed by the developing binary. Once distances are sufficiently large is that we start to see disks around either or both stars.

Such observations of primitive star systems tell us for example, that we shouldn't expect any planets around Alpha Centauri A or B stars, but on Proxima Centauri - which checks out so far as our telescopes are able to detect.

The dimensions of the protoplanetary or circumbinary disk in our binary system is quite important so we know what constraints the natural formation of planets and asteroid belts in our system.

As a general rule, the inner radius of the circumbinary disk is roughly 2 ~ 3 times that of the orbital seperation between the stars, and depending on the actual mass ration between the stars, a debris belt might be present in the inner system from material that was unnable to accrete into planets. BUT WATCH FOR THE MASS AND SEPARATION.

The example above highlights the need to give attention to either orbital separation or mass of the binaries in question. The case presented ends up being a sterile gas giant system because the star configuration pushes the stability zone way past the snow line of the binary (~1.2 AU).

This system can be made much more friendly to Earth-like planets or moons if the stars have greater mass, and thus more luminous output towards planets so far away. Or conserving the stars but increasing their orbital separation until we get a system around either or both stars, in which case it would be over 100~1000 AU wide.

It is possible to have both P-type and S-type planets in those intermediate distances, as long as the systems are relatively packed.

For an eccentricity of 0.2, the following is a good approximation for the outer limit of the star's planetary system:

Which results in larger limits the smaller the companion is, since its gravitational effect diminishes with mass, and the larger is the mass difference between stars, the more circular are the planets orbits around them. The planet's orbital eccentricity gets higher the more eccentric and similar are the parent stars, tending towards 1/3rd the eccentricity value of the parents as it approaches 0.5. So we would expect that the planets around our previous example to have a eccentricities of less than 0.06, which is actually more circular than the Moon's orbit around the Earth.

Given the luminosity of the stars in the example is 0.37 solar luminosities, if we want a planet on the circunstellar habitable zone at 0.6 AU with 2 AU to spare, then we have to space the stars such as that the Dsk_outer/separation value is equal to some 3 AU in this case, which ends up being 8% of the total separation by proportion, since both stars have the same mass. This gives us a minimum separation of 37 AU! If we get more conservative with the room to spare part, we easily arrive at separations far above 50 AU, which is confirmed while looking at binaries in space.

As for how far can circumbinary (P-type) planets orbit the binary, we can only estimate based on some real-life examples. COCONUTS-2b orbits its parent at distance of 6,000-7,000 AU, but remember that its system lies in deep interstellar space and might be easily disrupted by future star flybys. Given the mass of 6.3 Jupiters for the planet, and 0.37 solar masses (typical of cited M3 stars), the gravitational pull is close to 540,000 teranewtons, using F = GMm/R².

Referring to that value of 540,000 teranewtons as close to an upper limit, then the maximum distance we can put a similar planet around our binary pair is around to 14,100 AU. While the minimum distance would be above 3x the orbital separation, or greater than 110 AU, sadly too far into the depths of space for any life, but a P-type planet nonetheless. The vast distances involved for allowing P-type planets are also why they are such a rare find in nature.

Now that we've recollected everything essential into configurating such systems, let's talk about...

LAGRANGE POINTS

They are equipotential regions of space, where both the parent and satellite mass exert similar amounts of force over. 

These Lagrangian points exist between the two bodies at L1, opposite to the satellite at L2, opposite to the parent at L3, leading the orbit at L4 and trailing the orbit at L5.

In the solar system, the most notable lagrangian objects are the Trojan asteroids, which lie in the L4 and L5 points of the Sun-Jupiter system.

Now, because the "size" or "strength" of the Lagrange point is dependant on the system size and mass, the greater the mass we can shove into those points and get away with a stable system.

We have to note here that due the distances involved, the points L1, L2, and L3 are all too unstable for significant stay of any bodies, which is why we use them as gateways / low-effort insertion points for spacecraft, as you can quite easily travel through them. Points L4 and L5 are more forgiving for stability and degree of precision needed to hang around.

Going over planetary masses and doing a bit of equation balance, we find out that equation is only satisfied if the mass disparity for the primary body is gigantic, that is, if it holds 96% or more of the system's mass, which leaves us with 4% of the primary body mass to distribute amongst our satellite and Trojan-like body.

Back to the Sun-Jupiter system, the Sun being 1040x the mass of Jupiter - it easily comports at least another Jupiter-mass object at either lagrange points because of how extreme is the mass difference. Though such extreme cases are unlikely in nature, because of the way planets form and disturb nearby material during the formation process, hence why we have some asteroids with negligible mass as trojans, not whole planets.

T-TYPE  for 'TROJAN' PLANETS

For this to work out, we'd need a brown dwarf if our primary star is Sun-like, in which case, that brown-dwarf doesn't play a large role in illuminating the planet at all. If we want the secondary star be at least a red dwarf, then the primary star would need to be B7 blue giant star - that is also bad for us because blue stars are extremely short lived, just shy of 60-100 million years, enough to develop a primordial planet but no native life.

Say we want this system to live at least some 4.5 billion years, then our primary options are stars with up to 1.2 solar masses. That leaves us with up to 0.048 solar masses for a high-mass brown dwarf, low-key an actual flaring red dwarf (L0 or M9 type), with 1/1000th of the Sun's luminosity.

Located at either the L4 or L5 points of a double star system, such planets would have both suns fixed in the sky always at 60° apart, under the right conditions it is day in 2/3rds of the planet's surface at any point, and only truly night on the other 1/3rd.

But can we make it work? Uhhh... Kinda

My rendering of such hypothetical scenario, illumination is not realistic for viewing purposes

APPROACH 1: USE BROWN DWARFS

In this case, we give the planet a very slow rotational period, like +100 hours, this makes the planet have a very inefficient heat distribution, as when the night side turns back to the day side it had time to irradiate most of its energy. This is attainable in a three ways; the planet the is the tidally locked satellite of a gas giant, this way the orbital period of 4-ish days is the same as it's rotational period; or the planet has lots of moons, which slow the planet down over time; or the planet was struck in the deep past by another smaller planet, thus counter-acting its rotational momentum, or even, it has a slow retrograde rotation like Venus because of it.

We can also make this planet relatively small, so its volcanism and mass doens't make it retain a thick atmosphere, which would make this site unbearable due greenhouse effect.

And then we position such planet bit closer than what the habitable zone says it's safe, like 0.80 ~ 0.90x that distance.

The result is a world that gets near 1.5x as much sunlight as Earth (from the main star), but the thin atmosphere and amount of solar exposure would certainly be deadly to many known organisms. It might sound like a lot, given the Earth hasn't always had UV protection with ozone, but let's consider that the Earth's atmosphere has also been thicker in the deep past, but due atmospheric escape and gases becoming trapped in rocks, it has been getting thinner over time, which not only would have granted that Earth did not freeze in the Sun's early days as a main-sequence star, but also makes the planet habitable under the amount of sunlight we currently receive.

With a world getting that much sunlight from the start and having a thinner atmosphere with a slower or non-existent rock recycling process, we maximize the amount of radiation we can possibly get on the surface. The presence of liquid water on the surface also changes our outcome, since oceans are good heatsinks, the less water we get, the hotter it gets during the day, the colder are the nights. So we're looking for an alien world with a thin atmosphere and little to no water. This sounds pretty much in-line the large rocky moon of a gas giant in the habitable zone.

How large is this gas giant? Well, we cannot get away James Cameron style, with a small gas giant and it's unbelievably large moon. Gas planets are on average 10,000 to 40,000 times heavier than their moons, which means that a gas giant with an Earth-mass moon would need to be on the order of 4,000 to 40,000 Earth-masses, or at least 15 Jupiter masses. Even Saturn is 4,750x heavier than its largest moon, Titan - but for really large moons we have to get into the realm of Brown Dwarfs.

The brown dwarf aspect of the system also helps us to add more heat into the system, in which case, you wouldn't want to be under either the suns or the brown dwarf in the sky.

I suggest you too google the chosen names, cool trivia

Hekate, our moon of a trojan planet gets about 1.41x times our solar irradiation from its parent star - however, since it is only at 4 million km from its host brown dwarf, it finds itself tidally locked, receiving 1.41x times the energy we get from the sun, but most of it is in the form of infrared radiation instead of visible light and UV rays. So while the illumination of the planet is similar to that of Earth's, its infrared irradiation is nearly 4 times that received by the Earth.

The orbital period of Hekate is also about 11 days and 4 hours, which makes for 5 and half days in hot darkness, and 5 and half days in bright hotness.

The brown dwarf appears larger than the star in the sky, and would be its primary heat source.

The problems with this solution are:

1. Brown Dwarfs cool down over time. In this configuration, the system would only be overwhelmed with heat for the first few hundreds of million years after the system's formation, the brown dwarf would have cooled to a mostly inoffensive heat source at 500 K after 1 billion years, needless to say, the planet would be much more hospitable to life when older than that.
2. The planet rotation slows down over time. So we might get some "run away from the heat" action when the planet is primitive and young. But if the world is more mature, then the brown dwarf stays fixed in the sky as the planet tidally locks to it.
3. Extra heat is not the extra radition we're looking for. Of course, we want things to burn in the light of our evil star, but the extra heat is going to get distributed across the planet as the atmosphere spins, making such worlds a Venusian/Wet-Venusian by default.

How one experiences day and night cycles while tidally locked to a brown dwarf

Many bright stars hold companions which are much less luminous than themselves, it is harder to detect those around large bright stars than it is around dimmer less massive stars because of the greater effects of gravitational wobbling, hence the biases in data towards companions of large stars being generally heavy stellar remnants.

This type of system might actually exist in enough abundance as to cover several thousands of systems in the whole galaxy, for all single bright sun-like stars that's 110 candidates per 1000 stars. Even if the chances of this arrangement are 0.1% within this population, we're still looking at some 45 million systems across the Milky Way galaxy.

APPROACH 2: USE MORE STARS

We've previously calculated that even for a decently sized brown dwarf, our main star would have to be larger, and thus bluer, more luminous, and short-lived. So to circunvent this mass problem, we will not inflate a single star, but instead are more smaller stars as a center of mass.

My first example presented two 0.75 solar-mass stars, which amount to 1.5 solar masses, yet, the system is going to live for 25 billion years, and it is only 2/3rds as luminous as our Sun. Can we get away with that kind of arrangement? With that specific one, no, for already specified reasons that is rather very unlikely for planets to form that close to the binary pair as it is.

A modified version using near-solar mass twin stars for a total of 1.88 Msol would push the habitable zone outwards to a minimum distance where planets can form in stable orbits around binaries. Now, because of the distances involved, since the two main stars are so close together and far away from where we want our trojan planet, we will treat them as a single massive object with near double the mass of our Sun, pushing our 4% mass to work with up to 0.075 solar masses, which is inside the realm of red dwarfs even if we take a bit to feed a trojan brown dwarf. Interesting...

If we pack two sun-like stars 0.15 AU apart, then the next safest stable distance for another body would be around 2~3x that distance, at about 0.40 AU, where we could, put another star... or another pair of stars!

What I find interesting in this scenario is that we can get multiple eclipses in a row, or even, a multi-eclipse, because at certain astronomical alignments, more than 1 star can be eclipsed at once if the planet and star postions are just right.

Here is an attempt at showing how the eclipse shadows move as the stars move, notice that the planet zips by multiple shadows at once, the star's orbital plane is inclined 2.72 degrees from the brown dwarf's orbital plane.

You can make an animation like the previous one that by setting up a curve screen about the radius of your planet's/moon's orbit and let the sunlight projects shadows on it. In my case the whole thing spins because I've animated the whole orrery for accuracy.

I find the eclipse scenario quite enticing, as it poses a short window of safe surface activities before everyone needs to cover from intense sunlight. But here are some problems:

1. There isn't enough shadow time. The transit of a single eclipse under this configuration is ~13 hours, under the right conditons of inclination, the planet might experience a total of ~52 hours or ~2 days of eclipse per orbit, but typically over the year, the eclipses are far too spaced out, or if the shadows overlay we get darker but same-duration eclipses (assuming zero orbital inclination between planet and brown dwarf). Plus, the eclipses only happen across an arc that's 22.5° across the planet's orbit on the night-side of the brown dwarf, due the orbital separation of the stars and distance to this planet, that is only.
2. The lighting isn't radical enough. At a distance of 1.7 AU, the planet receives a collective 1.11x the solar constant from the parent stars, and an extra 0.92x all in infrared from the brown dwarf, to which it is likely tidally locked, the planet is also very likely to be a Venusian world.

The typical mass ratio in binary systems is 3:1, followed by 2:1 and 5:4, which sounds concerning at first. However, when in a multiple-star system, the tendency is for stars to settle with similar masses as opposed to unequal masses, followed then by a 3:2 ratio. So for this configuration, this system is not at all strange, however, our trojan scenario will be always a subset of those quadruple systems with equal mass, so we can confidently say that it is considerably less than 2 per 1000 stars, or about <<1 billion quadruple systems sporting sun-like components. We'd need to know how many of those systems have planets around them to narrow our subset, given we know at least 200 quadruple systems, from which we know only two that have planets (30 Ari, and Kepler-64 b), the chances drops down to about << 1% of those, that is a small subset of the less than 8 million quadruple systems with planets galaxy-wide. Pretty unlikely to occur in this exact configuration, though we get a lot of near-misses.

But not gonna lie, the dance of multiple stars is pretty hypnotizing to watch from a moving frame of reference...

IN THE NEXT POSTS WE EXPLORE OTHER WAYS TO MAKE CREMATORIA REAL

• S-type planets in close binaries
• Dying binaries
• Luminous black holes

- M. O. Valent, 05/02/2023